Chapter 3: Composition of Compounds and
Experimental Determination of Chemical Formulas
Section 3-1: Percent Composition by Mass
Section 3-2: Determination of Empirical and Molecular Formulas
Section 3-3: Experiment - Determining the Formula of a Hydrate
Chapter 3 Practice Exercises and Review Quizzes
Section 3-1: Percent Composition by Mass
We can determine the percent by
mass of a given element in a compound as follows:
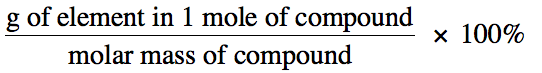
For example, to determine the
percent by mass of hydrogen in water, we divide the grams of hydrogen in 1 mole
of water by the molar mass of water:

Sample Exercise 3A:
Calculate the percent by mass of
carbon in (C2H5)2NH.
Solution:
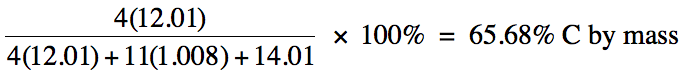
Section 3-2: Determination of Empirical and Molecular
Formulas
A molecular formula gives the actual number of atoms of each element
in one molecule of a compound. For
example, since a glucose molecule contains 6 carbon atoms, 12 hydrogen atoms,
and 6 oxygen atoms, the molecular formula of glucose is C6H12O6.
An empirical formula gives the simplest ratio of atoms of each element
in a compound. For example, since
the simplest ratio in glucose is 1 C atom: 2 hydrogen atoms: 1 oxygen atom, the
empirical formula of glucose is C1H2O1 or
simply CH2O. An empirical
formula also gives the simplest ratio of moles of each element in a
compound. Note, however, that an
empirical formula does NOT
give the simplest ratio of grams of each element in a compound.
The percent composition by mass of
an unknown compound can be determined by a variety of experimental methods,
after which the empirical formula of the unknown compound can be determined as
demonstrated in the problem below:
Sample Exercise 3B:
An unknown compound was found to be
55.77% carbon and 11.70% hydrogen by mass, with the remainder being
nitrogen. Determine the empirical
formula of the compound.
Solution:
First, subtract to obtain the
percent by mass of nitrogen in the compound:
100%
– 55.77% C – 11.70% H = 32.53% N
Next, assume you have exactly one
hundred grams of the unknown compound, in which case the percent by mass of
each element becomes equal to the grams of each element, and then convert grams
of each element to moles:
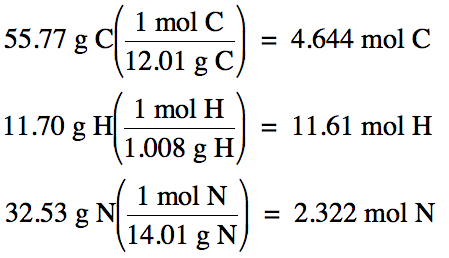
Recalling from the definition above
that an empirical formula gives the simplest ratio of moles of each element,
divide the moles of each element by the smallest number of moles in the group
and then round each result to the nearest whole number:
4.644 mol
C: 11.61 mol H: 2.322 mol N (divide each by 2.322)
= 2 mol C:
5 mol H: 1 mol N
Therefore,
the empirical formula of the unknown compound is C2H5N.
The molar mass of a compound can be
determined by a variety of experimental methods. If both the empirical formula and the molar mass of a compound
are known, the molecular formula of the compound can be determined as
demonstrated in the following problem:
Sample Exercise 3C:
A compound with empirical formula C2H5N
is found to have a molar mass of about 86 g/mol. What is the molecular formula of the compound?
Solution:
First, calculate the molar mass of
the empirical formula (EM):
EM = 2(12.01) + 5(1.008) + 14.01 = 43.07 g/mol
Next, divide the molar mass of the
compound given in the problem by the calculated molar mass of the empirical formula
and round the result to the nearest whole number:
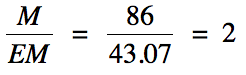
Finally,
multiply the subscripts in the empirical formula by the whole number above to
obtain the molecular formula:
C2x2H5x2N1x2
= C4H10N2 = molecular formula
Section 3-3: Experiment – Determining the
Formula of a Hydrate
A hydrate is an ionic compound with water
incorporated into the solid crystal.
For example, the hydrate MgSO4•7H2O contains 7
moles of water for every mole of MgSO4 in the crystal. The formula of a hydrate can be
determined experimentally by heating a known mass of the hydrate until all the
water is removed and then finding the mass of the remaining anhydrous
compound. The mass of water
removed can be obtained by subtraction, after which the calculated ratio of
moles of water to moles of the anhydrous compound yields the formula of the
hydrate, as demonstrated in the following problem:
Sample Exercise 3D:
To determine the value of x in the
formula of the hydrate BaCl2•xH2O, a student heated a
sample of the hydrate in an evaporating dish over a Bunsen burner to remove all
the water and recorded the following data:
|
Mass of
Empty Evaporating Dish |
22.36 g |
|
Mass of
Evaporating Dish + Hydrate |
32.23 g |
|
Mass of
Evaporating Dish + Anhydrous BaCl2 |
30.20 g |
Determine the value of x to the correct number of significant figures and the most likely formula
of the hydrate.
Solution:
First, subtract the mass of the
evaporating dish to find the mass of the hydrate before heating and the mass of
the anhydrous BaCl2 after heating:
32.23 g
– 22.36 g = 9.87 g hydrate before heating
30.20 g
– 22.36 g = 7.84 g anhydrous BaCl2 after heating
Next, subtract the results above to
find the mass of water removed by heating:
9.87 g
– 7.84 g = 2.03 g water removed
Note that we keep 2 decimal places
in all the masses calculated by subtraction above because all the original
measurements had 2 decimal places.
Finally, convert the masses of
water and BaCl2 to moles and find the ratio of moles of water to
moles of BaCl2:
M of H2O = 2(1.008) + 16.00 = 18.02 g/mol
M of BaCl2 = 137.3 + 2(35.45) = 208.2 g/mol
Note that we will keep an extra
sig. fig. (4 instead of 3) in the intermediate grams to moles calculations
below, but then round our final ratio to the correct number of sig. fig.s.
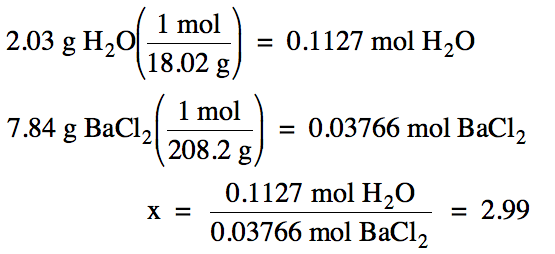
Based on the ratio calculated
above, there are most likely 3 moles of water for every mole of BaCl2
in the hydrate. Therefore, the
most likely formula of the hydrate is BaCl2•3H2O.
Note that the calculated ratio in
hydrate experiments may not be a whole number due to experimental error. For example, if the hydrate is underheated and not all the water is removed, the final
mass recorded will be too high. As
a result, the mass of the anhydrous compound found by subtraction will be too
high and the mass of water found by subtraction will be too low, leading to an
erroneously low ratio of moles of water to moles of anhydrous compound.
Chapter 3 Practice Exercises and Review Quizzes:
3-1) Calculate the percent by mass
of oxygen in Ca3(PO4)2.
Click for Solution
3-1)
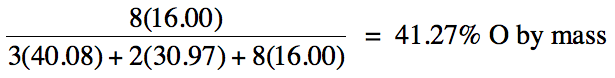
3-2) What
is the empirical formula of C6H12O3?
Click for Solution
3-2) divide
all subscripts by 3 to get simplest ratio = C2H4O =
empirical formula
3-3) (a)
An unknown compound was found to be 26.17% chlorine and 56.10% fluorine by
mass, with the remainder being carbon.
Determine the empirical formula of the compound.
(b) In a separate
experiment, the molar mass of the unknown compound was found to be about 271
g/mol. Determine the molecular
formula of the compound.
Click for Solution
3-3) (a)
100% - 26.17% Cl – 56.10% F = 17.73% C by mass
Assume one hundred grams of unknown
compound:
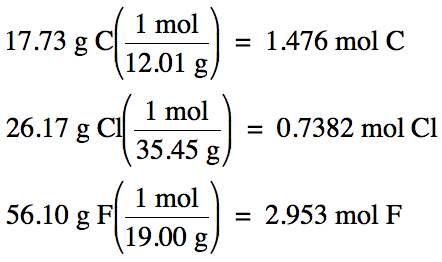
1.476 mol
C: 0.7382 mol Cl: 2.953 mol F (divide each by 0.7382)
= 2 mol C:
1 mol Cl: 4 mol F
Therefore,
empirical formula is C2ClF4.
(b) EM = 2(12.01) + 35.45 + 4(19.00) = 135.5 g/mol
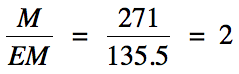
C2x2Cl1x2F4x2
= C4Cl2F8 = molecular formula
3-4) To determine the value of x in
the formula of the hydrate CaSO4•xH2O, a student heated a
sample of the hydrate in an evaporating dish over a Bunsen burner to remove all
the water and recorded the following data:
|
Mass of
Empty Evaporating Dish |
19.73 g |
|
Mass of
Evaporating Dish + Hydrate |
21.50 g |
|
Mass of
Evaporating Dish + Anhydrous CaSO4 |
20.80 g |
Determine the value of x to the correct number of significant figures and the most likely formula
of the hydrate.
Click for Solution
3-4)
21.50 g
– 19.73 g = 1.77 g hydrate before heating
20.80 g
– 19.73 g = 1.07 g anhydrous CaSO4 after heating
1.77 g
– 1.07 g = 0.70 g water removed
M of CaSO4 = 40.08 + 32.07 + 4(16.00) =
136.2 g/mol

Based on the ratio calculated
above, there are most likely 5 moles of water for every mole of CaSO4
in the hydrate. Therefore, the
most likely formula of the hydrate is CaSO4•5H2O.
