Chapter 20: Nuclear Chemistry
Section 20-1: Balancing Nuclear Equations
Section 20-2: Nuclear Kinetics and Half-Life
Chapter 20 Practice Exercises and Review Quizzes
Section 20-1: Balancing Nuclear Equations
During a nuclear reaction, the
nuclei of the isotopes involved will undergo a change in composition. Each particle involved in a nuclear
reaction will be represented as follows, where Z is the atomic number and A is
the mass number:
![]()
When a nuclear reaction is
balanced, the sum of the Z values for the reactants must equal the sum of the Z
values for the products. Likewise,
the sum of the A values for the reactants must equal the sum of the A values
for the products. Note that
charges are typically omitted when nuclear reactions are written.
Types of radioactive decay include:
A. Alpha decay (or alpha emission)
= nucleus of an isotope X emits a helium-4 nucleus to produce a new isotope
Y. The equation is balanced as
follows:

B. Beta decay (or beta emission) =
nucleus of an isotope X emits a particle equivalent to an electron to produce a
new isotope Y. The equation is
balanced as follows:
![]()
C. Positron decay (or positron
emission) = nucleus of an isotope X emits a particle equivalent to an electron,
but with a positive charge, to produce a new isotope Y. The equation is balanced as follows:
![]()
D. Electron capture = electron from
an inner orbital is absorbed into the nucleus of isotope X to produce a new
isotope Y. The equation is
balanced as follows:
![]()
In each case above, the identity of
the isotope Y can be determined by matching the atomic number of Y with an
element on the periodic table:
Sample Exercise 20A:
Write balanced equations for the
following nuclear reactions:
a. Uranium-235 decays by alpha
emission.
b. Rubidium-87 decays by beta emission.
c. Potassium-38 decays by positron
emission.
d. Iron-55 decays by electron
capture.
Solution:
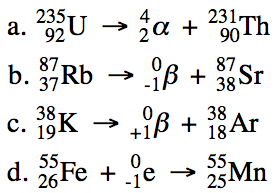
A nuclear reaction may also be
initiated by bombarding a sample of an isotope with particles such as neutrons
or protons in order to produce a new isotope. Other particles may be produced in the process as well. The symbols for a neutron and a proton
are as follows:
![]()
Sample Exercise 20B:
Neutron bombardment of nitrogen-14
produces a proton and a new isotope.
Write a balanced equation for this nuclear reaction.
Solution:
![]()
Section 20-2: Nuclear Kinetics and Half-Life
ln Qf = -kt + ln Qi
The half-life (t1/2) of
a radioactive isotope is the time required for the isotope to decay to half its
initial quantity. Using the
equation above, we can derive the relationship between half-life and the rate
constant k by substituting 0.5Qi for Qf and t1/2 for t:
ln (0.5Qi) = -kt1/2 + ln Qi
kt1/2 =
ln (Qi /0.5Qi) = ln 2
t1/2 =
ln 2/k = 0.693/k and k = 0.693/t1/2
Replacing k with 0.693/t1/2 in the equation ln Qf =
-kt + ln Qi, we obtain the
following equation:
ln Qf = -(0.693/t1/2)t + ln Qi
Sample Exercise 20C:
The half-life of bromine-80 is 18
minutes. What mass of bromine-80
will remain if a 96 gram sample decays for 25 minutes?
Solution:

If the equation ln Qf = -(0.693/t1/2)t
+ ln Qi is solved for t,
we obtain:
t = (t1/2/0.693)ln (Qi/Qf)
Sample Exercise 20D:
The half-life of potassium-40 is
1.25 x 109 years. How
much time is required for a 9.48 mol sample of potassium-40 to decay to 1.22
mol?
Solution:

We can also solve for t1/2 as follows:
Sample Exercise 20E:
A sample of radon-222 containing
6.54 x 1025 atoms requires 17.6 days to decay to 2.66 x 1024 atoms. Calculate the half-life of
radon-222.
Solution:

Chapter 20 Practice Exercises and Review Quizzes:
20-1) Write balanced equations for
the following nuclear reactions:
a. Lead-196 decays by electron
capture.
b. Phosphorus-28 decays by positron
emission.
c. Radium-226 decays by alpha
emission.
d. Zinc-73 decays by beta
emission.
Click for Solution

20-2) Proton bombardment of
magnesium-26 produces an alpha particle and a new isotope. Write a balanced equation for this
nuclear reaction.
Click for Solution

20-3) The half-life of oxygen-13 is
0.00870 seconds. How many moles of
oxygen-13 will remain if a 38.4 mol sample decays for 0.0125 seconds?
Click for Solution

20-4) The half-life of iodine-131 is 8.04 days. How much time is required for a sample containing 6.56 x 1023 iodine-131 atoms to decay to 1.26 x 1023 atoms?
Click for Solution

20-5) A 48 gram sample of
magnesium-28 requires 69 hours to decay to 5.0 grams. Calculate the half-life of magnesium-28.
Click for Solution

